Marilyn vos Savant is an American writer whose birth name was “Marilyn Mach” — as in, “Marilyn (descendant of physicist Ernst) Mach.” As one might expect given her ancestry, she’s smart. In 1986, the Guinness Book of World Records listed her with a record-high IQ of 228, which made her a niche celebrity. That same year, she leveraged her intelligence and fame into a Sunday “Ask Marilyn” column in Parade magazine in which (even to this day) she solves all sorts of puzzles posed by readers. On September 9, 1990, she answered a question that has come to be known as “The Monty Hall Problem.” In response to her explanation, about 10,000 people wrote to Parade, adamantly saying that vos Savant was wrong. About 1,000 of these people had PhDs. But vos Savant — a college dropout — was right.
This is the 25th installment of The Labyrinthian, a series dedicated to exploring random fields of knowledge in order to give you unordinary theoretical, philosophical, strategic, and/or often rambling guidance on daily fantasy sports. Consult the introductory piece to the series for further explanation.
The Monty Hall Problem: The 1990-91 Version of “What Color Is This Dress?”
After the mass outcry, vos Savant wrote a follow-up column, explaining her answer further. That only caused more mass outcry. She wrote two more columns devoted to the problem. Even more mass outcry. Very quickly, the Monty Hall problem became something that people debated in public — sort of like the “What Color Is This Dress?” debate of 2015 — and on July 21, 1991, The New York Times finally stepped in and published a front-page feature article in which vos Savant was vindicated.
And yet even then some people didn’t believe that she was right. Paul Erdős, a very accomplished mathematician, didn’t accept vos Savant’s answer until he had seen a computer simulation playing out (thousands of times) the scenario presented in the Monty Hall problem. For some reason, people simply did not want to believe that her answer was right.
What precisely is the Monty Hall problem? Here’s the exact question that Craig F. Whitaker of Columbia, Maryland, posed to vos Savant:
Suppose you’re on a game show, and you’re given the choice of three doors. Behind one door is a car, behind the other, goats. You pick a door, say No. 1, and the host, who knows what’s behind the doors, opens another door, say No. 3, which has a goat. He says to you, “Do you want to pick door No. 2?” Is it to your advantage to switch your choice of doors?”
The question was popularly called the Monty Hall problem after the host of the TV show Let’s Make a Deal, in which Hall would present contestants with queries identical to the one above: “You’ve picked door 1, I’ve shown you what’s behind door 3 — and it’s not the car — so now do you want to stick with door 1 or switch to door 2?”
The answer is so simplistically obvious that it’s maddening, right?
It doesn’t matter whether you stay with door 1 or switch to door 2, because at this point between the two doors the odds are exactly 50/50. Clearly!
Except they’re not.
What’s Behind Door No. 1 Is Probably A Goat
Before you call me an idiot — which I am, but for reasons totally unrelated to this issue — let me show you a simple diagram proving that that one should always switch doors:
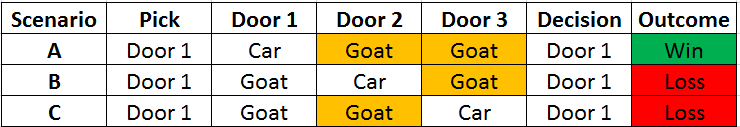
Let me walk you through each of the potential scenarios according to the question that vos Savant originally answered. And in these scenarios, let’s assume that the host is ALWAYS going to open a door to reveal a goat, because that’s what actually happens on scenarios like this when presented on a TV show. Why would the host open a door, reveal a car to you, and then say, “Behind these two remaining doors are two goats. Do you want to switch doors?”
Scenario A: The car is behind Door 1, and the choice is Door 1
You choose Door 1, and the host opens either Door 2 or Door 3. In this scenario it doesn’t matter which one he chooses since a goat is behind either. You choose to stick with Door 1. The host opens Door 1. The CAR! Congratulations!!! You just won a CAR!!!!!!
Aren’t you glad that you didn’t switch doors?
Scenario B: The car is behind Door 2, and the choice is Door 1
You choose Door 1, and the host opens Door 3 — because he must open Door 3 — because out of Doors 2 and 3 he must open the door that has a goat behind it. You choose to stick with Door 1. The host opens Door 1. It’s a f***ing goat. Congratulations. You just won a f***ing goat.
Don’t you wish that you would’ve switched doors?
Scenario C: The car is behind Door 3, and the choice is Door 1
You choose Door 1, and the host opens Door 2 — because he must open Door 2 — because out of Doors 2 and 3 he must open the door that has a goat behind it. You choose to stick with Door 1. The host opens Door 1. It’s a f***ing goat. Again. Congratulations. You just won a f***ing goat. Again.
Don’t you wish that you would’ve switched doors? Again.
Side note: In my articles, “f***ing” stands for “failing.” It’s just that, to me, “fail” is a vulgar, four-letter word. It shouldn’t be used in polite company. Rarely should it be printed.
Anchoring (Again)
In the last Labyrinthian, I applied psychologist Daniel Kahneman’s idea of anchoring to PGA DFS. It made my boss sick — so I’ll stick with Door 1, know what I mean?
Essentially, anchoring is what happens when people get ideas, numbers, etc., in their heads and then make decisions impacted by (or anchored to) the information that is in their heads. I believe that the concept of anchoring can be applied to the Monty Hall problem in a number of ways. I also believe that the Monty Hall problem is applicable to DFS, which I’ll get to soon. (By the way, Bryan Mears has already applied Monty Hall to DFS. Our points are slightly different. You should read his piece.)
Perhaps I’ve become anchored to the idea of anchoring and so I have a skewed perspective — but I think it applies to the Monty Hall problem through this fact: When presented with the option to switch doors, most people choose not to switch doors. Why? If most people believe that the odds are 50/50, what reason do they have for not switching?
Perhaps some people — despite believing that the odds are even — also believe that they have selected the right door . . . even though they must also know that the odds of their having originally selected the correct door are only slightly better than 33 percent. These people have become anchored to their door. More to the point, they have become anchored to their prior decision.
Other people know that the odds of their choosing a wrong door at the start are almost 67 percent, but once a goat door has been opened they might think that the odds have shifted to 50/50 and then anchor to that belief. In effect, they anchor to the idea that their initial likely incorrectness no longer matters.
Finally, other people might anchor to a particular loser mindset informed by personal experience and the regrets from which they suffer. They think about the two potential negative outcomes. If they switch, the car could be behind the original door. Or if they don’t switch, the car could be behind the second door.
Almost regardless of the odds, these people would prefer to live with regret that stems from the latter outcome but not the former. They don’t want to put themselves in the position of being able to blame themselves for actively making a bad decision — and so they do nothing. Anchored to this risk-averse mentality of mediocrity, these people somehow forget that doing nothing is also a decision. And, in this case, it’s a bad one.
The F***ure to Act on New Information
Regardless of what they anchor to and why they err, everyone who decides not to switch doors in the Monty Hall problem ultimately makes the mistake of failing to evaluate the game’s updated information properly. They fail to understand the way in which they can leverage the new knowledge that they have. They believe that the manner in which the game has played out so far lends itself equally to the outcomes of winning and losing. They are drastically wrong.
Knowing where one of the goats is located benefits contestants only if they are willing to consider deeply the possibility that they originally picked the wrong door. But for some reason, even in games that involve randomness, people don’t want to consider the possibility (even the likelihood) that their original decisions might have been incorrect or might no longer apply to the updated set of circumstances. In general, people err in overestimating the odds of their initial accuracy.
Additionally — and this is something that few people appreciate — knowing the location of a goat and correctly taking that information into account actually make people likely to win the car. Based on the rules of the game and how it plays out, the game itself is structured to benefit people who switch.
Unlike almost any game that exists, because of the knowledge that one gains during the contest, one is always likely (from the very beginning) to be a winner — as long as one is willing to evaluate in-contest information and make in-game adjustments based on that information. And, by that same token, one is always likely to lose if one does not make sound in-game evaluations and adjustments.
The Monty Hall problem is ultimately about people’s inability or unwillingness to act on new and beneficial information once a contest has commenced.
DraftKings’ Late Swap
In the way that DraftKings structures its contests, they are remarkably comparable to the Monty Hall problem. Whereas on FanDuel one is unable to act on new in-game information since all lineup spots lock when contests start, on DK because of the late-swap feature one can and should use in-game information to make lineup adjustments.
Almost all DFS players know about the late swap capabilities on DK. Some people sense that the biggest game feature distinguishing DK and FD is late swap. Fewer players use late swap regularly. And even fewer recognize this simple truth — on DK, the people who use late swap are likely to win and the people who don’t swap are likely to lose.
Jonathan Bales has written definitively on late swap in the past. In one article, he argues that one can strategically use late swap to assume or decrease risk and to add or remove stacks when necessary. In another piece, Bales argues that one can use late swap in order to be aggressive in early games and to hedge a position in later games. Many ways exist to use late swap. The important thing is to be mindful of it — to construct your lineups in such a way so as to maximize its utility.
But so many people don’t think about late swap when building their lineups, and I’m not talking just about the standard technique of putting the player with the latest game in the flex position — which, by the way, everyone should do. Rather, one should apply this “late-game flex” mentality to all positions. When constructing a lineup, one should use as many players from later games as possible (all things being equal) in order to give oneself enhanced late swap flexibility.
I’m not saying that you should put suboptimal late-game players in your lineup merely so that you can pivot away from them later. I am saying, though, that if a player in a late game is equal to a player in an earlier game, you should choose the player who will provide your lineup with greater late-swap flexibility.
The Panopticon
Michel Foucault was a French philosopher and cultural theorist who was very influential in academia in the 1970s and 1980s. After his death in 1984, Foucault’s work continued to be widely read by graduate students across a number of fields well into the 2000s. Even today, his legacy is large. In 1975, he published Surveiller et punir, a book in which he explores the development of theories and techniques of interrogation, criminalization, judgment, torture, imprisonment, and execution in France. In English, the title of this book was translated as Discipline and Punish.
In the book, Foucault considers at length the Panopticon — an idea created and designed by English philosopher and lawyer Jeremy Bentham in the last decades of the 1700s. Essentially, the Panopticon is a circular prison with a guard tower in the middle. In this environment, all inmates are capable of being observed and yet because of the position of the tower they are not able to see if they actually are observed at any given moment. And from his vantage point the watchmen has the capability to observe any prisoner he chooses for as long as he chooses, collecting an unlimited amount of data on that person.
For Foucault, the Panopticon was the ultimate symbol of knowledge as power. For the watchman, the ability to have full knowledge of all prisoners was a constitutive component of his power. And, for the prisoners, their inability to see the watchman or know if they were being observed was a core part of their impotence.
In DFS, knowledge is power. Using late swap enables you to leverage as much information as possible. Building your lineup into a Panopticon so that you can observe games as they unfold and benefit from the continued collection of data is vitally important.
One of my first Labyrinthian articles was on Sun Tzŭ and the five sins a DFS general can commit. Can you imagine a general not making in-battle adjustments once he realized that his initial plans were no longer applicable? Similarly, can you imagine the coach of a professional sports team not making halftime adjustments?
Door 1: If you’re not using late swap on DK, you probably shouldn’t play on DK. Instead, you should play exclusively on FD or another site that doesn’t enable late swap and on which you won’t be disadvantaged.
Door 2: And yet, if you aren’t playing on DK and maximizing your late swap potential, then you maybe shouldn’t play DFS at all, since you are deciding to forgo one of the biggest DFS edges available to you.
Door 3: Just play on DK and use late swap so that you can discipline and punish those who don’t. Win the cash equivalent of a car. Become the GOAT. Anchor your existence to winning. Be a savant.
Put a bow on that b*tch.
Read: “batch”
———
The Labyrinthian: 2016, 25
Previous installments of The Labyrinthian can be accessed via my author page. If you have suggestions on material I should know about or even write about in a future Labyrinthian, please contact me via email, Matthew@FantasyLabs.com, or Twitter @MattFtheOracle.